



Next: Friction
Up: Dynamics
Previous: Dynamics
Contents
Kinetic Analysis
The force analysis will be performed with the free body diagram presented in figure 3.1.
Figure 3.1: Free Body Diagram.
The figure on the left shows the forces involved. The figure on the right shows the resulting accelerations.
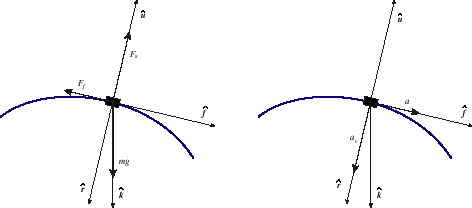
|
The car is treated as a point mass, which rides a distance  above the rails. The forces on
the car are described below. Recall that there will be no lateral force.
above the rails. The forces on
the car are described below. Recall that there will be no lateral force.
- Friction Force
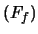
- The friction force always opposes the movement of the
car along the track, acting in the
 direction. The components of the friction force are
explained in more detail in section 3.1.1.
direction. The components of the friction force are
explained in more detail in section 3.1.1.
- Seat Force
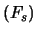
- The seat force acts perpendicular to the forward direction of travel. This direction is referred to as the up direction,
 , so named because it is what the passenger may perceive as ``up'' while navigating the coaster. It is the direction directly out of the top of the car, away from the seat. The up vector determines the orientation of the car around the forward vector. It is specified such that there will be no lateral acceleration, and is calculated such that the non-forward component of gravity, combined with the centripetal force, lies in the up direction.
, so named because it is what the passenger may perceive as ``up'' while navigating the coaster. It is the direction directly out of the top of the car, away from the seat. The up vector determines the orientation of the car around the forward vector. It is specified such that there will be no lateral acceleration, and is calculated such that the non-forward component of gravity, combined with the centripetal force, lies in the up direction.
- Force of Gravity
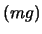
- Gravity always acts toward the earth, the
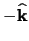 direction.
direction.
The acceleration of the car is composed of the following two quantities:
- Forward Acceleration

- The forward tangential acceleration acts in the
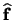 direction.
direction.
- Centripetal Acceleration
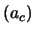
- The centripetal acceleration is calculated as
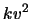 . It acts toward the center of curvature, in the radial
. It acts toward the center of curvature, in the radial
 direction.
direction.
The up and radial vectors are necessarily perpendicular to the forward vector, although in general
they are not parallel to each other.
The following can be derived from the free body diagram.
Subsections




Next: Friction
Up: Dynamics
Previous: Dynamics
Contents
Darla Weiss
2000-02-13
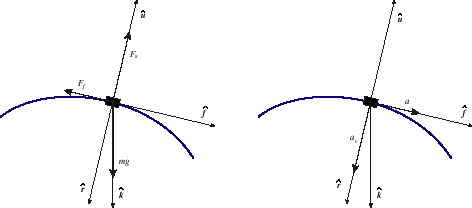
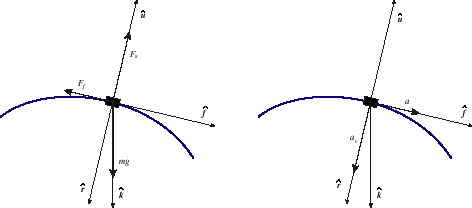
![]() above the rails. The forces on
the car are described below. Recall that there will be no lateral force.
above the rails. The forces on
the car are described below. Recall that there will be no lateral force.