Circular Region: $(a_{1}t_{1_{f}} \leq s_{proj} \leq a_{1}t_{1_{f}} + r \delta )$

The vertical angle at any point on the circular region is determined with the following.

$$\phi = \gamma_{1} + \frac{s_{proj} - a_{1}t_{1_{f}}}{r}$$

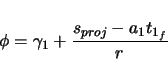
The end of the first clothoid occurs when $t=t_{1_{f}}$. The position at $t=t_{1_{f}}$ is found, and the center of the circular region is determined from it. This point, $(x_{c},z_{c})$, can be seen in figure 2.5.

$$\begin{eqnarray*} x_{c} & = & a_{1} \ensuremath\int_{0}^{t_{1_{f}}} \cos(\frac{... ...frac{\pi}{2} u^{2})\,du - r \cos \left( \frac{\delta}{2} \right) \end{eqnarray*}$$

The positions in the circular region are then determined with the following.

$$x = x_{c} + r \sin\phi$$ (2.63)

$$y = s_{proj} \tan\alpha$$ (2.64)

$$z = z_{c} + r \cos\phi$$ (2.65)

Derivatives with respect to arclength will need to be taken to determine the remaining functions. Since the position vector is expressed in terms of $\phi$, the chain rule is applied.

$$\begin{eqnarray*} \ensuremath \frac{d\phi}{ds} & = & \ensuremath \frac{d\phi}{d... ...nsuremath \frac{ds_{proj}}{ds} \\ & = & \frac{1}{r} \cos\alpha \end{eqnarray*}$$

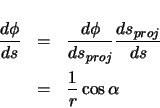
The forward vector is calculated as the derivative of the position vector with respect to arclength.

$$f_{x} = \ensuremath \frac{d\left( x_{c} + r \sin\phi \right)}{d\phi} \ensuremath \frac{d\phi}{ds}$$

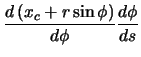$$= \cos\phi \cos\alpha$$ (2.66)

$$f_{y} = \ensuremath \frac{d(s_{proj} \tan\alpha)}{ds_{proj}} \ensuremath \frac{ds_{proj}}{ds}$$

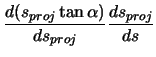$$= \sin\alpha$$ (2.67)

$$f_{z} = \ensuremath \frac{d\left( z_{c} + r \cos\phi \right)}{d\phi} \ensuremath \frac{d\phi}{ds}$$

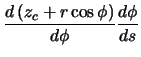$$= \sin\phi \cos\alpha$$ (2.68)

The radial vector is calculated as the derivative of the forward vector with respect to arclength.

$$\begin{eqnarray*} r_{x} & = & \ensuremath \frac{d\left( \cos\phi \cos\alpha \ri... ... \frac{d\phi}{ds} \\ & = & \frac{1}{r} \cos\phi \cos^{2}\alpha \end{eqnarray*}$$

The unit vector in the direction of the radial vector must be determined.

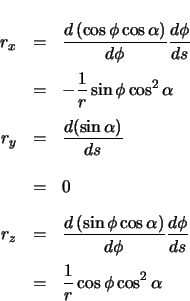
$$r_{x} = -\sin\phi$$ (2.69)

$$r_{y} = 0$$ (2.70)

$$r_{z} = \cos\phi$$ (2.71)

The curvature is the magnitude of the (non-unit) radial vector.

$$k = \frac{1}{r} \cos^{2}\alpha$$ (2.72)