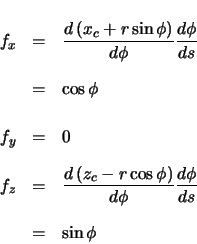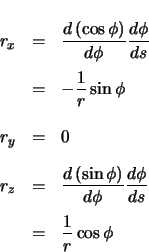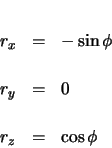Next: Clothoid 2:
Up: Joint Elements
Previous: Clothoid 1:
Contents
The vertical angle at any point on the circular region is determined with the following.
The circular region is first calculated as if the joint began from horizontal. The end of the first
clothoid occurs when  . The center of the circular region can be found from the
following.
. The center of the circular region can be found from the
following.
The positions within the circular region are then determined as shown.
If a downward joint is being calculated,  is negated. The circular region is then rotated
is negated. The circular region is then rotated
 radians to line it up with adjacent regions. See figure 2.11.
radians to line it up with adjacent regions. See figure 2.11.
Derivatives with respect to arclength will need to be taken to determine the remaining functions.
Since the position vector is expressed in terms of  , the chain rule is applied.
, the chain rule is applied.
The forward vector is calculated by taking the derivative of the position vector as it was first
calculated. It will then be transformed in the same way as the position vector was.
The  coordinate is negated if a downward joint is being calculated. The vector is then rotated
coordinate is negated if a downward joint is being calculated. The vector is then rotated  radians about the
radians about the  axis.
axis.
The radial vector is calculated as the derivative of the forward vector (with respect to arclength)
as it was initially calculated.
The unit vector in the direction of the radial vector must be determined.
The  coordinate is negated if a downward joint is being calculated. The vector is then rotated
coordinate is negated if a downward joint is being calculated. The vector is then rotated
 radians about the
radians about the  axis. The result will still be a unit vector, as rotation does not
alter magnitude.
axis. The result will still be a unit vector, as rotation does not
alter magnitude.
The curvature is the magnitude of the (non-unit) radial vector.
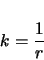 |
(2.136) |




Next: Clothoid 2:
Up: Joint Elements
Previous: Clothoid 1:
Contents
Darla Weiss
2000-02-13
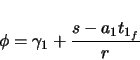
![]() . The center of the circular region can be found from the
following.
. The center of the circular region can be found from the
following.
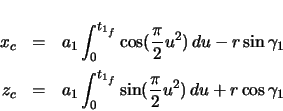
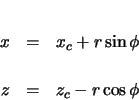
![]() , the chain rule is applied.
, the chain rule is applied.